How to Find the Sum and Average of Elements in an Array in JavaScript

If you need to compute the sum or the average of an array of elements in JavaScript, you can use reduce to get the shortest possible solution:

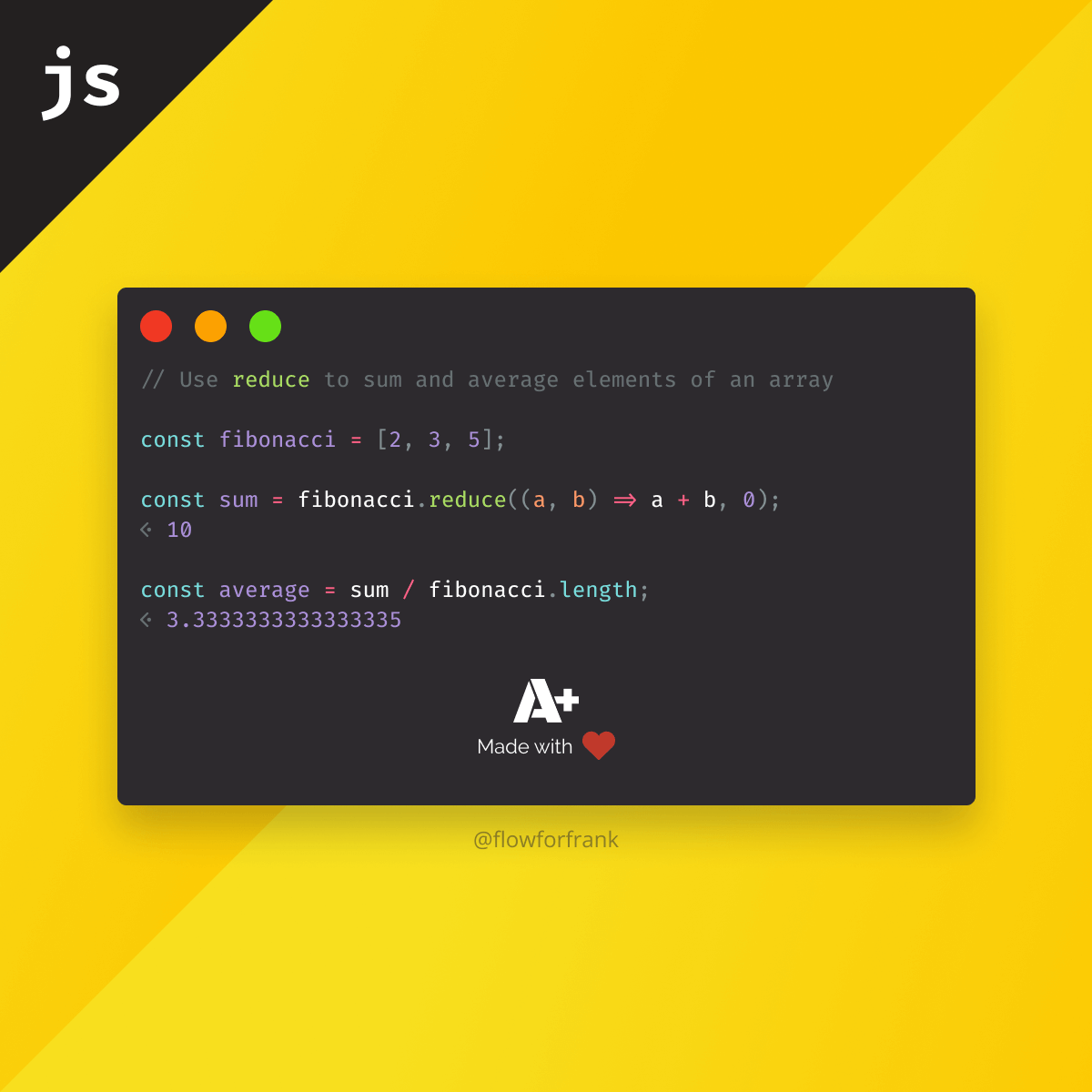
const fibonacci = [2, 3, 5];

// Will return 10
const sum = fibonacci.reduce((a, b) => a + b, 0);

// Will return 3.3333333333333335
const average = sum / fibonacci.length;

If you are unsure whether the elements of the array are numbers, you can also cast them to one, using the built-in Number function:

const sum = fibonacci.reduce((a, b) => (Number(a) + Number(b)) || 0, 0);

Get access to 300+ webtips 💌

Level up your skills and master the art of frontend development with bite-sized tutorials.

We don't spam. Unsubscribe anytime.

Get Access to Webtips

---

Explanation

Let's break this code down line-by-line so we fully understand how does it work. We start with this line:

fibonacci.reduce((a, b) => a + b, 0);

The reduce function takes in two arguments:

• A reducer function that you can define, that results in a single output for the element. This function has two mandatory arguments:
  • The accumulator, denoted by a. This is the accumulated value returned from the last invocation of the callback.
  • The current value, denoted by b, that is being processed in the array.
• Optionally, you can also provide an initial value that will be used as the first argument to your callback. In the example above, we provided an initial value of 0.

With the example array, these are the values of each argument, step by step:

[2, 3, 5].reduce((0, 2) => 0 + 2, 0); // First iteration  -> returns 2
[2, 3, 5].reduce((2, 3) => 2 + 3, 0); // Second iteration -> returns 5
[2, 3, 5].reduce((5, 5) => 5 + 5, 0); // Third iteration  -> returns 10

And to find the average, we can divide the sum by the number of numbers. In the second example, when we were unsure about whether we deal with numbers, we have the following:

fibonacci.reduce((a, b) => (Number(a) + Number(b)) || 0, 0);

But why do we need the || 0 at the end? This is because if either a or b cannot be converted to a number, the return value will be NaN. NaN + NaN, or NaN + any number will be returned as NaN, and we don't want that, so instead, we fall back to 0.

✅ Number('1') + Number('2') -> 1 + 2 -> 3
🔴 Number('a') + Number('b') -> NaN + NaN -> NaN

50 JavaScript Interview QuestionsAnd their answers explainedHere are 50 JavaScript interview questions that often come up during a technical interview. In one place, one resource to learn from.Learn More

• Documentation for Array.prototype.reduce - MDN
• The Number function - MDN